Managing Wind Pool Risk with Portfolio Optimization
Jun 18, 2012
Editor's Note: Previous AIRCurrents articles explored AIR's portfolio optimization solutions for reinsurers and insurers. This time, Matthew Holland discusses how insurers can use optimization to improve wind pool decision-making.
Wind pools are a market of last resort for property owners unable to buy property insurance through the traditional market. Private insurers share risk with wind pools based on assessments. Assessments are impacted by premium and property locations voluntarily written by private insurers. In a wind pool area (usually coastal counties), the more an insurer writes voluntarily, the smaller the assessments, and vice versa.
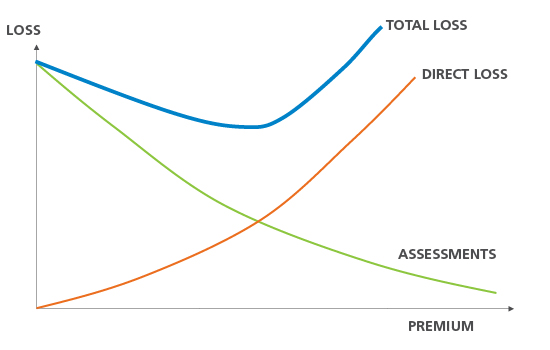
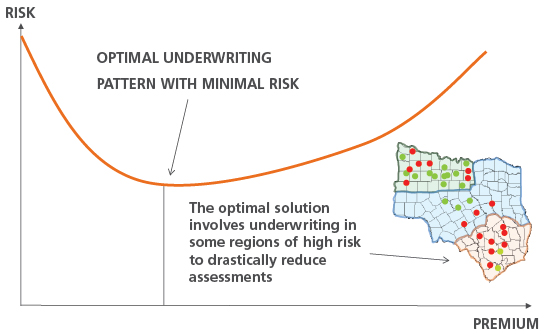
Writing business in states with wind pools offers insurers opportunities for profitable growth, but also exposes them to direct losses from voluntarily written policies and wind pool assessments. The general relationship between direct losses and assessments and the impact on total losses is shown in Figure 1. Insurers have less control over assessment risk than direct risk because they do not control the underwriting, risk mitigation, reinsurance purchasing, and claims settlement practices of the wind pool. Insurers can "write out" of the assessment risk by voluntarily writing enough wind pool eligible policies to reduce their assessments to zero. However, wind pool rates can be inadequate for insurers and conflict with profitable growth. Alternatively, insurers can leave coastal wind policies for the wind pool, but this increases the wind pool size and the insurer's risk associated with assessments. The challenge is to find an optimum level of underwriting in the wind pool area to minimize the total risk from direct loss and assessments.
By: Matthew Holland, CCM
Edited by Nan Ma, CCM
The article: Discusses how an optimization process improves an insurer's risk management within a wind pool.
Highlights: Wind pools create a business environment of competing objectives and multiple dependencies. Optimal underwriting guidelines are found by balancing company goals, implementation constraints, wind pool rates, and assessments. The optimization process incorporates sensitivity testing and adapts to changes in the business environment.
Defining the Problem Using an Optimization Framework
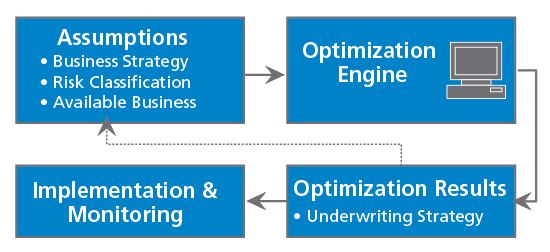
The general optimization problem is to maximize a goal subject to a set of constraints. Obtaining clear definition of goals and constraints can be challenging. An optimization framework defines goals such as maximizing profitable growth or minimizing risk while considering constraints such as preserving credit rating, maintaining key relationships, abiding by regulations, and developing implementable underwriting guidelines. Catastrophe model output, premiums, exposure management, reinsurance costs, investment income, and other non-catastrophe considerations are incorporated into the framework where appropriate.
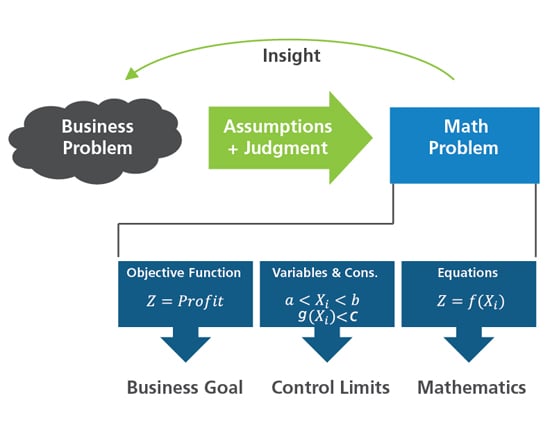
In the case of managing wind pool risk, there are many interconnected and moving pieces. Company-specific goals and constraints increase the complexity. The optimal underwriting pattern depends on premium and direct losses resulting from the insurer's voluntary underwriting plus assessments. The framework defines the wind pool assessments, treatment of direct and assessment losses, and an appropriate solution space of underwriting patterns. Once the problem is defined, optimization algorithms efficiently search the solution space.
Evaluating Enterprise Risk
For this article, assume that the insurer's goal is to minimize enterprise-wide risk, which the insurer has defined as the tail value at risk (TVaR) at the 1.00% exceedance probability level. Thus, the objective function is to minimize TVaR for the insurer's entire business, and the insurer will alter their wind pool area underwriting decisions to satisfy the objective function.
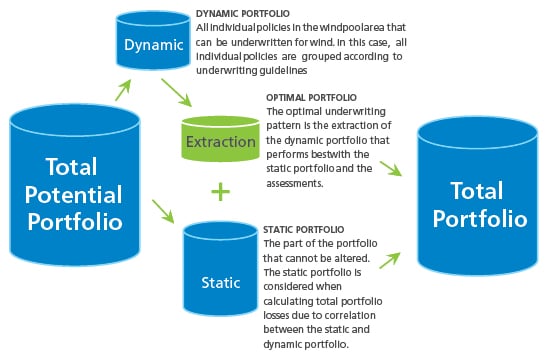
Figure 3 shows the insurer's total portfolio under consideration, which has two components—the static portfolio, which is everything in the insurer's total countrywide portfolio not in the wind pool area and thus not subject to change in the optimization exercise and an extraction from the dynamic portfolio, which is all policies in the wind pool area that can be voluntarily underwritten for wind and thus subject to inclusion or exclusion based on the optimization. Because the choice to underwrite new business will be based on a set of defined underwriting criteria, in order to maximize computational efficiency in the optimization, the individual policies in the dynamic portfolio are grouped according to underwriting guidelines. The decision variable is the choice to write or not write each policy group in the dynamic portfolio. The policy groups written are extracted from the dynamic portfolio and added to the total portfolio.
Direct losses from the static portfolio and the dynamic portfolio extraction are combined to obtain total portfolio losses to the enterprise. The static portfolio is included to account for correlation between the static (countrywide) and dynamic (wind pool area) portfolios. The total portfolio direct loss (extraction loss plus static loss) is combined with assessments to obtain total loss. The optimal underwriting pattern is the extraction portfolio that minimizes the TVaR of total losses.
The Optimal Solution Is in the Form of Implementable Underwriting Guidelines
Optimization results need to align with business reality and should be easy to justify internally and externally. In the dynamic portfolio, policies are grouped using the risk classification system insurers use to manage their business. The underwriting decision for each policy group is the underwriting guideline for all individual policies with the characteristics of the policy group. Policies are grouped by characteristics, including:
- Hazard attributes, such as distance to coast and/or elevation.
- Vulnerability categories based on building characteristics, such as construction/occupancy classes, year-built bands, mitigation features or some combination of these various features. AIR can provide guidance on a grouping strategy for the risks under consideration.
- Agent networks or geography, such as county, ZIP Code, or grid cell.
- Premium buckets.
- Other classifications used for assessment purposes, such as territory or line of business.
Table 1 shows an example of how individual policies can be grouped according to the characteristics of an insurers risk classification system. Each row in Table 1 represents one unique policy group. For example, Policy Group 1 is the aggregation of all individual policies with distance to coast of less than or equal to 1 mile, has low vulnerability as defined by building characteristics, located in County 1, and high relative premium rates.
| Policy Group Identifier | Distance to Coast | Vulnerability Category | County Identifier | Premium Bucket | Underwriting Decision: Write/Don't Write |
|---|---|---|---|---|---|
| 1 | ≤1 mile | Low | 1 | High | ? |
| 2 | ≤1 mile | Low | 1 | Low | ? |
| 3 | ≤1 mile | Medium | 1 | High | ? |
| 4 | ≤1 mile | Medium | 1 | Low | ? |
| 5 | >1 mile | High | 2 | High | ? |
| 6 | >1 mile | High | 2 | Low | ? |
Policy groups can vary based on the sensitivity of specific policies. Detailed policy groups can be used for sensitive coastal policies and less-sensitive policies can be grouped using simpler categories. Sensitive policies can be grouped based on finer resolutions of vulnerability such as multiple construction/occupancy categories, and/or finer geographic resolution such as one square mile grid cells. At the finest resolution, the most sensitive policies are evaluated on an individual policy basis.
Grouping policies aligns optimization with the end business goal. Policy-grouping reduces computation time, makes the solution more practical and intuitive, and provides insight about what characteristics make a policy more or less desirable for an insurer's business.
Wind Pool Assessment Modeling Challenges
Wind pool risk varies throughout the United States. Similar to a private insurer, wind pools have unique underwriting, capital management, and risk transfer goals that vary based on the geography and political climate in which they write. Modeling wind pool assessments is similar to modeling participation in a wind pool's reinsurance program, with the exception that participation in assessments varies based on the insurer and the wind pool's underwriting and premium patterns. Additionally, premium and loss treatment in assessment functions can vary by location and line of business.
In a wind pool area, an insurer's assessment participation can change based on the decision to voluntarily write wind for an individual policy. An insurer's pricing and underwriting pattern impacts voluntary premium, which impacts assessment participation. For example, with pricing held constant, assessments can change based on underwriting changes. Similarly, holding underwriting decisions constant, assessments can change based on pricing changes. The underwriting pattern impacts the insurer's direct losses and impacts the wind pool's exposure, losses, and total assessments. When an insurer writes fewer policies, the potential assessments of all companies grow and the insurer-specific participation in the increased assessments grows. Because of these relationships, an insurer's assessments are calculated dynamically within the optimization based on each underwriting pattern tested.
Problem Formulation
The more an insurer writes voluntarily, the larger the direct loss and the smaller the assessments to that insurer. The less an insurer writes voluntarily; the smaller the direct loss and the larger the assessments. To minimize risk from both direct loss and assessments constitutes an optimization problem. The optimization problem is to minimize enterprise risk (objective function), as measured by TVaR, by controlling the underwriting pattern (decision variables). Given a realizable and adequate fixed premium pattern, the problem is formally stated as:
| Minimize: | ||
|---|---|---|
| TVaR(L) | objective function | |
| Subject to: | ||
| L = D + A | total loss function | |
| D = E + S | direct loss function | |
| A = A(Xi, Pvi, Pw, W) | assessment participation function | |
| E = ∑(Xi*Gi) | extraction portfolio loss function | |
| Xi ∈ {0,1} | policy group underwriting constraint | |
| Where: | ||
| TVaR(L) = total portfolio TVaR function | Pw = Pw(Xi): wind pool written premium | |
| Xi = i'th policy group underwriting decision | S = static portfolio loss (constant) | |
| Gi = i'th policy group loss | W = wind pool loss | |
| Pvi = realizable and adequate insurer voluntary premiums for the i'th policy group | ||
Realizable and Adequate Pricing
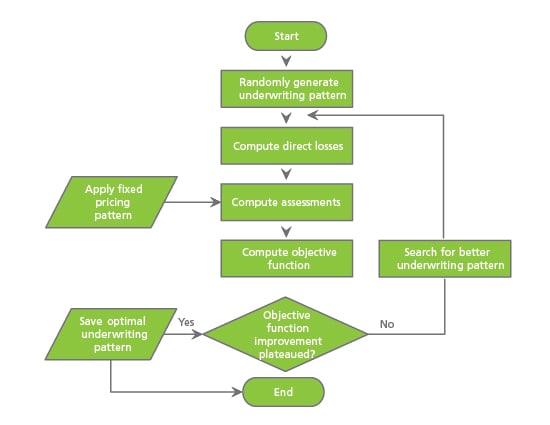
Wind pool rates can be below adequate and insurers do not want to write unprofitable policies solely to minimize total risk from direct losses and assessments. The higher premium realized, the more likely that rates are adequate and the greater the reduction in assessments. Figure 4 shows an optimization flowchart assuming a fixed pricing pattern. The flowchart is summarized as follows:
- Randomly generate underwriting pattern.
- For the underwriting pattern:
- Add extraction portfolio losses to static portfolio losses to compute direct losses for the total portfolio.
- Apply pricing pattern to determine premium and exposure for both the insurer and wind pool. Determine wind pool losses and compute assessments according to the assessment participation function.
- Add assessments to direct losses to compute total losses.
- Compute the objective function (TVaR of total losses) and evaluate the underwriting pattern.
- Search for better underwriting patterns until improvement in the objective function plateaus.
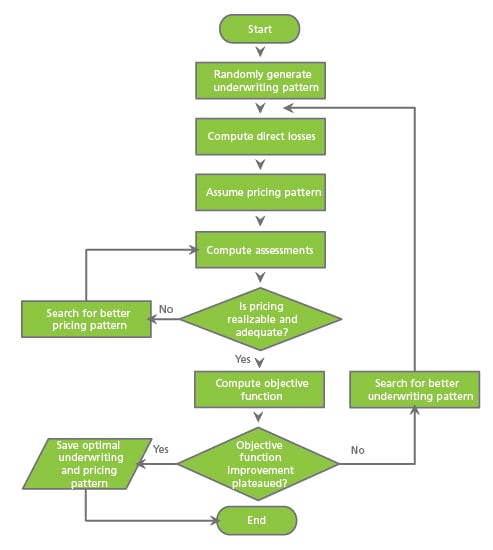
Pricing pattern assumptions must be realizable in a competitive market. Insurers may determine that realizable rates are equal to wind pool rates, or equal to wind pool rates plus a premium load. Finding an optimal pricing pattern is complex because of the recursive relationship between pricing and assessments. Pricing adequacy depends on assessments, assessments depend on the pricing and the underwriting pattern. Figure 5 shows how to address the relationship between pricing and assessments. Premium is tested according to what is realizable and adequate in a competitive market. The output is the optimal combination of underwriting pattern and pricing pattern.
With a flexible and repeatable optimization engine, insurers can assess how results change with different pricing assumptions. Pricing assumptions can be based on the wind pool's rates, an insurer's current rates, or optimal pricing based on adequacy and market-competitive rates.
Optimization Results, What-If Scenarios, and Sensitivity Testing
Wind pool optimization answers the question, "What is the optimal way to write business in a wind pool?" The optimization process, interpretation of results, and alternative analyses expose risks and opportunities. The optimal underwriting guidelines can reveal which risk-classifiers explain wind risk the best. Figure 6 shows that the insurer's business is sensitive to writing too little premium and it is safer to write more than the optimal amount.
Results are analyzed in three phases:
Phase 1: Interpreting and validating the optimal solution
Phase 2: Comparing the current underwriting pattern to the optimal solution in phase 1
Phase 3: Analysis of alternatives, sensitivities, and what-if scenarios
The optimal solution is a benchmark to quantify tradeoffs between alternatives. The framework and engine support optimization of alternative scenarios and deterministic testing in phases 2 and 3. Phase 1 may motivate testing of a similar, but qualitatively preferable, solution. Insurers may learn they can close 80% of the gap between their current state and the optimal state with 20% of the portfolio changes between current and optimal. Figure 7 illustrates how the optimal solution is used as a benchmark compared to alternatives.
A flexible process provides insurers with the ability to adapt to changes in business strategy, market prices, and wind pool exposure and regulations. What-if scenarios and uncertainty testing result in robust business strategies. What-if scenarios help evaluate alternative policy groups, pricing assumptions, and risk and return metrics. Sensitivities to consider include:
- A blend between AIR modeled catastrophe losses and alternative views of risk
- Warm sea surface temperature catalogs and/or alternative storm surge assumptions
- Wind pool changes such as:
- Uncapped assessments when catastrophe losses exceed wind pool catastrophe coverage
- Surplus depletion following catastrophic seasons, which increases the potential assessments for future events (for example, TWIA's financial resources were drastically reduced after Hurricane Ike in 2008)
A near-optimal underwriting pattern in multiple scenarios might be preferable to an underwriting pattern that is optimal in one scenario, but mediocre in alternative scenarios. Evolving markets, regulatory environments, and new business objectives are continually reassessed and incorporated into the optimization process. The process creates actionable solutions that fit current business needs and are flexible enough to promote profitable long-term growth.
Closing Thoughts
Optimization helps determine the best strategy to satisfy business goals and constraints in an environment with competing objectives and multiple sources of uncertainty. AIR's wind pool optimization framework simplifies a complex problem into manageable components. Advanced analytics combined with catastrophe, insurance, and software expertise results in an optimization process that can be permanently adopted into a company's workflow. The process results in insight into the problem, an implementable solution, and the knowledge and tools for continuous optimization.
